|
The acousto-optic
effect allows controlling any parameters of an optical wave: amplitude,
phase, frequency and polarization. Therefore, different modifications
of the acousto-optic modulators can be realized. Besides, it should be
taken into account that acousto-optic interaction makes it possible to
introduce information into an optical beam by means of both temporal and
spatial modulation.
At the present time, the temporal modulators of light intensity are the
most widespread. These modulators employ a progressive acoustic wave modulated
in amplitude by an informative signal. The principle of operation of the
modulators is based on the dependence of the diffracted light intensity
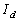 on the acoustic power on the acoustic power
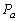 . A scheme of the modulator
operating in the Bragg regime of diffraction is shown in Fig. 1m. Electrical
oscillations of a frequency . A scheme of the modulator
operating in the Bragg regime of diffraction is shown in Fig. 1m. Electrical
oscillations of a frequency  from a HF generator
(1) are modulated in amplitude by an informative signal from a HF generator
(1) are modulated in amplitude by an informative signal 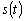 and then enter a piezotransducer
(2) of an acousto-optic cell (3). The regime of travelling acoustic waves
is provided by an acoustic absorber (4). The carrier frequency and then enter a piezotransducer
(2) of an acousto-optic cell (3). The regime of travelling acoustic waves
is provided by an acoustic absorber (4). The carrier frequency 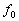 is chosen equal to
the central frequency of the transducer. Modern technology permits attaining
the transducer bandwidth is chosen equal to
the central frequency of the transducer. Modern technology permits attaining
the transducer bandwidth  as great as as great as  ; so that ; so that 
A basic characteristic of the modulator is the modulation bandwidth 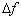 . It is obvious that . It is obvious that
 cannot be greater
than cannot be greater
than 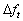 . However, there exist
additional restrictions of the modulation band defined by peculiarities
of acousto-optic interaction. For the modulators, the parameter . However, there exist
additional restrictions of the modulation band defined by peculiarities
of acousto-optic interaction. For the modulators, the parameter  is of considerable
importance, where is of considerable
importance, where  and and 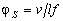 are the optical and
acoustic beam divergences, are the optical and
acoustic beam divergences,  is the optical wavelength
in vacuum, n is the refractive index, v is the acoustic velocity, f is the acoustic frequency, d and l
are the optical and acoustic beam widths in the acousto-optic interaction
plane. If the condition is the optical wavelength
in vacuum, n is the refractive index, v is the acoustic velocity, f is the acoustic frequency, d and l
are the optical and acoustic beam widths in the acousto-optic interaction
plane. If the condition  is satisfied, the
modulation bandwidth is determined by the relationship is satisfied, the
modulation bandwidth is determined by the relationship 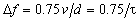 . This expression
shows that in the limit . This expression
shows that in the limit 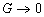 the modulation band
is defined exclusively by the time the modulation band
is defined exclusively by the time  required for ultrasound to cross the optical beam.
With increasing the parameter G the modulation
band becomes narrower because of volume effects in acousto-optic interaction.
In this case the ratio required for ultrasound to cross the optical beam.
With increasing the parameter G the modulation
band becomes narrower because of volume effects in acousto-optic interaction.
In this case the ratio 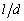 plays the decisive
role. plays the decisive
role.
The optimization of modulator parameters is conventionally produced with
taking into consideration both the modulation band and the consumed power.
Such optimization leads to the following value of the parameter G:
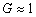 which defines the
condition of optical and acoustic beam divergences matching. In this case which defines the
condition of optical and acoustic beam divergences matching. In this case
 . . |
(1m) |
If the diffraction efficiency  does not exceed 30%,
the consumed acoustic power does not exceed 30%,
the consumed acoustic power 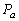 can be calculated
from the equation can be calculated
from the equation
 , , |
(2m) |
where
M is the acousto-optic figure of merit,
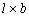 is the cross-section
of the acoustic beam. Eqs (1m) and (2m) enable defining optimal parameters
of the modulating acousto-optic cell. Supposing the acoustic bandwidth is the cross-section
of the acoustic beam. Eqs (1m) and (2m) enable defining optimal parameters
of the modulating acousto-optic cell. Supposing the acoustic bandwidth
 to given, one can
yield: to given, one can
yield:
 |
(3m) |
Here the reasonable assumptions  and and  are taken into account.
Graphic results of the calculations for are taken into account.
Graphic results of the calculations for  and for different
acousto-optic materials are presented in this section. and for different
acousto-optic materials are presented in this section.
|